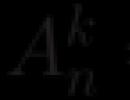Middle line t. Trapeze. Properties, features, area. The middle line of the trapezoid - materials for preparing for the exam in Mathematics. Property of the bisector of a trapezoid

A quadrilateral with only two parallel sides is called trapeze.

The parallel sides of a trapezoid are called its grounds, and those sides that are not parallel are called sides. If the sides are equal, then such a trapezoid is isosceles. The distance between the bases is called the height of the trapezoid.

Middle line of the trapezium

The median line is a segment connecting the midpoints of the sides of the trapezoid. The midline of a trapezoid is parallel to its bases.

Theorem:

If a line intersecting the midpoint of one side is parallel to the bases of a trapezoid, then it bisects the second lateral side trapezoid.

Theorem:

The length of the midline is equal to the arithmetic mean of the lengths of its bases

MN || AB || DC
AM=MD; BN=NC

MN midline, AB and CD - bases, AD and BC - sides

MN=(AB+DC)/2

Theorem:

The length of the midline of a trapezoid is equal to the arithmetic mean of the lengths of its bases.

The main task: Prove that the midline of a trapezoid bisects a segment whose ends lie in the middle of the bases of the trapezoid.

Middle Line of the Triangle

The line segment connecting the midpoints of the two sides of a triangle is called the midline of the triangle. It is parallel to the third side and its length is half the length of the third side.
Theorem: If a line intersecting the midpoint of one side of a triangle is parallel to the other side of the given triangle, then it bisects the third side.

AM = MC and BN = NC =>

Applying Triangle and Trapezoid Midline Properties

The division of a segment into a certain number of equal parts.
Task: Divide segment AB into 5 equal parts.
Solution:
Let p be a random ray whose origin is point A and which does not lie on line AB. We sequentially set aside 5 equal segments on p AA 1 = A 1 A 2 = A 2 A 3 = A 3 A 4 = A 4 A 5
We connect A 5 to B and draw lines through A 4 , A 3 , A 2 and A 1 that are parallel to A 5 B. They intersect AB at B 4 , B 3 , B 2 and B 1 respectively. These points divide segment AB into 5 equal parts. Indeed, from the trapezoid BB 3 A 3 A 5 we see that BB 4 = B 4 B 3 . In the same way, from the trapezoid B 4 B 2 A 2 A 4 we get B 4 B 3 = B 3 B 2

While from the trapezoid B 3 B 1 A 1 A 3 , B 3 B 2 = B 2 B 1 .
Then from B 2 AA 2 it follows that B 2 B 1 = B 1 A. In conclusion, we get:
AB 1 = B 1 B 2 = B 2 B 3 = B 3 B 4 = B 4 B
It is clear that in order to divide the segment AB into another number of equal parts, we need to project the same number of equal segments onto the ray p. And then continue in the manner described above.

middle line figures in planimetry - a segment connecting the midpoints of the two sides of a given figure. The concept is used for the following figures: triangle, quadrilateral, trapezium.

Encyclopedic YouTube

1 / 3

✪ Grade 8, Lesson 25, The middle line of the triangle

✪ geometry MIDDLE LINE OF TRIANGLE Atanasyan Grade 8

✪ The middle line of the triangle | Geometry 7-9 grade #62 | info lesson

Subtitles

Middle line of the triangle

Properties

the midline of a triangle is parallel to the base and equal to half of it.
at the intersection of all three middle lines, 4 equal triangles are formed, similar (even homothetic) to the original one with a coefficient of 1/2.
the middle line cuts off a triangle that is similar to the given one, and its area is equal to one fourth of the area of the original triangle.
The three middle lines of the triangle divide it into 4 equal (identical) triangles similar to the original triangle. All 4 such identical triangles are called medial triangles. The central one of these 4 identical triangles is called the complementary triangle.

signs

if the segment is parallel to one of the sides of the triangle and connects the midpoint of one side of the triangle with a point on the other side of the triangle, then this is the midline.

Middle line of the quadrilateral

Middle line of the quadrilateral A line segment that joins the midpoints of opposite sides of a quadrilateral.

Properties

The first line connects 2 opposite sides. The second connects 2 other opposite sides. The third one connects the centers of the two diagonals (not in all quadrilaterals the diagonals are bisected by the intersection point).

If in a convex quadrilateral the midline forms equal angles with the diagonals of a quadrilateral, then the diagonals are equal.
The length of the midline of a quadrilateral is less than or equal to half the sum of the other two sides if these sides are parallel, and only in this case.
The midpoints of the sides of an arbitrary quadrilateral are the vertices of a parallelogram. Its area is equal to half the area of the quadrilateral, and its center lies at the point of intersection of the median lines. This parallelogram is called the Varignon parallelogram;
The last point means the following: In a convex quadrilateral, four middle lines of the second kind. Middle lines of the second kind- four segments inside the quadrangle passing through the midpoints of its adjacent sides parallel to the diagonals. Four middle lines of the second kind convex quadrilateral cut it into four triangles and one central quadrilateral. This central quadrilateral is a parallelogram of Varignon.
The point of intersection of the midlines of the quadrilateral is their common midpoint and bisects the segment connecting the midpoints of the diagonals. In addition, she is

Your privacy is important to us. For this reason, we have developed a Privacy Policy that describes how we use and store your information. Please read our privacy policy and let us know if you have any questions.

Collection and use of personal information

Personal information refers to data that can be used to identify or contact a specific person.

You may be asked to provide your personal information at any time when you contact us.

The following are some examples of the types of personal information we may collect and how we may use such information.

What personal information we collect:

When you submit an application on the site, we may collect various information, including your name, phone number, email address, etc.

How we use your personal information:

Collected by us personal information allows us to contact you and inform you about unique offers, promotions and other events and upcoming events.
From time to time, we may use your personal information to send you important notices and messages.
We may also use personal information for internal purposes such as auditing, data analysis and various studies to improve the services we provide and to provide you with recommendations regarding our services.
If you enter a prize draw, contest or similar incentive, we may use the information you provide to administer such programs.

Disclosure to third parties

We do not disclose information received from you to third parties.

Exceptions:

In the event that it is necessary - in accordance with the law, judicial order, in legal proceedings, and / or based on public requests or requests from state bodies in the territory of the Russian Federation - disclose your personal information. We may also disclose information about you if we determine that such disclosure is necessary or appropriate for security, law enforcement, or other public interest reasons.
In the event of a reorganization, merger or sale, we may transfer the personal information we collect to the relevant third party successor.

Protection of personal information

We take precautions - including administrative, technical and physical - to protect your personal information from loss, theft, and misuse, as well as from unauthorized access, disclosure, alteration and destruction.

Maintaining your privacy at the company level

To ensure that your personal information is secure, we communicate privacy and security practices to our employees and strictly enforce privacy practices.

In this article, another selection of tasks with a trapezoid has been made for you. Conditions are somehow connected with its middle line. Task types are taken from the open bank of typical tasks. If you wish, you can refresh your theoretical knowledge. The blog has already covered tasks whose conditions are associated with, as well as. Briefly about the middle line:

The middle line of the trapezoid connects the midpoints of the sides. It is parallel to the bases and equal to their half-sum.

Before solving problems, let's consider a theoretical example.

Given a trapezoid ABCD. Diagonal AC intersecting with the midline forms a point K, diagonal BD a point L. Prove that the segment KL is equal to half the difference of the bases.

Let's first note the fact that the midline of a trapezoid bisects any segment whose ends lie on its bases. This conclusion suggests itself. Imagine a segment connecting two points of the bases, it will split this trapezoid into two others. It turns out that a segment parallel to the bases of the trapezoid and passing through the middle of the side on the other side will pass through its middle.

It is also based on the Thales theorem:

If on one of the two straight lines several equal segments are sequentially laid aside and parallel lines are drawn through their ends, intersecting the second straight line, then they will cut off equal segments on the second straight line.

That is, in this case K is the middle of AC and L is the middle of BD. Hence EK is the middle line triangle ABC, LF is the midline of triangle DCB. According to the property of the midline of a triangle:

We can now express the segment KL in terms of bases:

Proven!

This example is not just given. In tasks for independent solution there is such a task. Only it does not say that the segment connecting the midpoints of the diagonals lies on the midline. Consider the tasks:

27819. Find the midline of a trapezoid if its bases are 30 and 16.

We calculate by the formula:

27820. The midline of the trapezoid is 28 and the smaller base is 18. Find the larger base of the trapezoid.

Let's express the larger base:

In this way:

27836. A perpendicular dropped from the apex of an obtuse angle to the greater base of an isosceles trapezoid divides it into parts having lengths 10 and 4. Find the midline of this trapezoid.

In order to find the middle line, you need to know the bases. The base AB is easy to find: 10+4=14. Find DC.

Let's construct the second perpendicular DF:

Segments AF, FE and EB will be equal to 4, 6 and 4 respectively. Why?

In an isosceles trapezoid, the perpendiculars dropped to the larger base divide it into three segments. Two of them, which are the legs of cut off right-angled triangles, are equal to each other. The third segment is equal to the smaller base, since when constructing the indicated heights, a rectangle is formed, and in the rectangle, the opposite sides are equal. In this task:

Thus DC=6. We calculate:

27839. The bases of the trapezoid are in ratio 2:3, and the midline is 5. Find the smaller base.

Let's introduce the coefficient of proportionality x. Then AB=3x, DC=2x. We can write:

Therefore, the smaller base is 2∙2=4.

27840. The perimeter of an isosceles trapezoid is 80, its midline is equal to the lateral side. Find the side of the trapezoid.

Based on the condition, we can write:

If we denote the middle line through x, we get:

The second equation can already be written as:

27841. The midline of the trapezoid is 7, and one of its bases is 4 more than the other. Find the larger base of the trapezoid.

Let's denote the smaller base (DC) as x, then the larger one (AB) will be equal to x + 4. We can record

We got that the smaller base is early than five, which means that the larger one is equal to 9.

27842. The midline of the trapezoid is 12. One of the diagonals divides it into two segments, the difference of which is 2. Find the larger base of the trapezoid.

We can easily find the larger base of the trapezoid if we calculate the segment EO. It is the middle line in triangle ADB, and AB=2∙EO.

What do we have? It is said that the middle line is equal to 12 and the difference between the segments EO and OF is equal to 2. We can write down two equations and solve the system:

It is clear that in this case it is possible to select a pair of numbers without calculations, these are 5 and 7. But, nevertheless, we will solve the system:

So EO=12–5=7. Thus, the larger base is equal to AB=2∙EO=14.

27844. In an isosceles trapezoid, the diagonals are perpendicular. The height of the trapezoid is 12. Find its midline.

Immediately, we note that the height drawn through the intersection point of the diagonals in an isosceles trapezoid lies on the axis of symmetry and divides the trapezoid into two equal rectangular trapezoids, that is, the bases of this height are divided in half.

It would seem that in order to calculate the average line, we must find the grounds. Here a small dead end arises ... How, knowing the height, in this case, calculate the bases? And no how! Many such trapezoids with a fixed height and diagonals intersecting at an angle of 90 degrees can be built. How to be?

Look at the formula for the midline of a trapezoid. After all, we do not need to know the bases themselves, it is enough to know their sum (or half-sum). This we can do.

Since the diagonals intersect at right angles, isosceles right triangles are formed with height EF:

It follows from the above that FO=DF=FC, and OE=AE=EB. Now let's write down what the height expressed through the segments DF and AE is equal to:

So the middle line is 12.

* In general, this is a problem, as you understand, for an oral account. But I'm sure the detailed explanation provided is necessary. And so ... If you look at the figure (provided that the angle between the diagonals is observed during construction), the equality FO=DF=FC, and OE=AE=EB immediately catches your eye.

As part of the prototypes, there are also types of tasks with trapezoids. It was built on a sheet in a cell and it is required to find the middle line, the side of the cell is usually 1, but there may be another value.

27848. Find the midline of the trapezoid ABCD if the sides of the square cells are 1.

It's simple, we calculate the bases by cells and use the formula: (2 + 4) / 2 = 3

If the bases are built at an angle to the cell grid, then there are two ways. For example!

The median line of a trapezoid, and especially its properties, are very often used in geometry to solve problems and prove certain theorems.

is a quadrilateral with only 2 sides parallel to each other. Parallel sides are called bases (in Figure 1 - AD And BC), the other two are lateral (in the figure AB And CD).

Median line of the trapezoid- this is a segment connecting the midpoints of its lateral sides (in Figure 1 - KL).

Properties of the midline of a trapezoid

Proof of the trapezium midline theorem

Prove that the midline of a trapezoid is equal to half the sum of its bases and parallel to these bases.

Dana trapezoid ABCD with midline KL. To prove the properties under consideration, it is required to draw a straight line through the points B And L. In figure 2, this is a straight line BQ. And also continue the base AD to the intersection with the line BQ.

Consider the resulting triangles LBC And LQD:

By definition of the midline KL dot L is the midpoint of the segment CD. It follows from this that the segments CL And LD are equal.
∠BLC = ∠QLD because these angles are vertical.
∠BCL = ∠LDQ, since these angles are crosswise lying at parallel lines AD And BC and secant CD.

From these 3 equalities it follows that the triangles considered earlier LBC And LQD are equal on 1 side and two angles adjacent to it (see Fig. 3). Consequently, ∠ LBC = ∠LQD, BC=DQ and the most important thing - BL=LQ => KL, which is the midline of the trapezoid ABCD, is also the midline of the triangle ABQ. According to the property of the midline of a triangle ABQ we get.