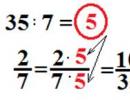How to find the angle in a trapezoid from the sides. Angles of an isosceles trapezoid. Properties of a trapezoid circumscribed about a circle

A trapezoid is a geometric figure, a quadrilateral that has two parallel lines. The other two lines cannot be parallel, in which case it would be a parallelogram.

Types of trapezoids

There are three types of trapezoids: rectangular, when two angles of the trapezoid are 90 degrees; equilateral, in which the two lateral lines are equal; versatile, where the lateral lines are of different lengths.

Working with trapezoids, you can learn to calculate their area, height, line size, and also figure out how to find the angles of a trapezoid.

Rectangular trapezoid

A rectangular trapezoid has two 90 degree angles. The sum of the remaining two angles is 180 degrees. Therefore, there is a way to find the angles of a right-angled trapezoid, knowing the size of one of the angles. Let it be, for example, 26 degrees. You just need to subtract the sum of the known angles from the total sum of the angles of the trapezoid - 360 degrees. 360-(90+90+26) = 154. The desired angle will be 154 degrees. It can be considered simpler: since two angles are right angles, then in total they will be 180 degrees, that is, half of 360; the sum of oblique angles will also be equal to 180, so you can calculate easier and faster 180 -26 = 154.

Isosceles trapezoid

An isosceles trapezoid has two equal sides that are not bases. There are formulas that explain how to find the angles of an isosceles trapezoid.

Calculation 1, if the dimensions of the sides of the trapezoid are given

They are designated by the letters A, B and C: A are the dimensions of the sides, B and C are the dimensions of the base, smaller and larger, respectively. The trapezoid should also be called ABCD. For calculations, it is necessary to draw the height H from angle B. A right triangle BNA is formed, where AN and BH are the legs, AB is the hypotenuse. Now you can calculate the size of the leg AN. To do this, it is necessary to subtract the smaller one from the larger base of the trapezoid and divide in half, i.e. (с-b)/2.

To find the acute angle of a triangle, you need to use the cos function. Cos of the desired angle (β) will be equal to a / ((c-b)/2). To find out the size of angle β, you need to use the arcos function. β = arcos 2a/c-b. Because two angles of an equilateral trapezoid are equal, then they will be: angle BAD = angle CDA = arcos 2a/c-b.

Calculation 2. If the dimensions of the bases of the trapezoid are given.

Having the values of the bases of the trapezoid - a and b, you can use the same method as in the previous solution. From angle b it is necessary to lower the height h. Having the dimensions of the two legs of the triangle we just created, you can use a similar trigonometric function, only in this case it will be tg. To convert an angle and get its value, you need to use the arctg function. Based on the formulas, we obtain the dimensions of the required angles:

β = arctg 2h/s-b, and angle α = 180 - arctg 2h/s-b/

Regular scalene trapezoid

There is a way to find the larger angle of a trapezoid. To do this, you need to know the dimensions of both acute angles. Knowing them, and knowing that the sum of the angles at any base of a trapezoid is 180 degrees, we conclude that the required obtuse angle will consist of the difference of 180 - the size of the acute angle. You can also find another obtuse angle of the trapezoid.

Trapezoid problems do not seem difficult in a number of shapes that have been studied previously. A rectangular trapezoid is considered as a special case. And when searching for its area, sometimes it is more convenient to divide it into two already familiar ones: a rectangle and a triangle. You just have to think a little, and you will definitely find a solution.

Definition of a rectangular trapezoid and its properties

An arbitrary trapezoid has parallel bases, and the sides can have arbitrary angles to them. If we consider a rectangular trapezoid, then one of its sides is always perpendicular to the bases. That is, two angles in it will be equal to 90 degrees. Moreover, they always belong to adjacent vertices or, in other words, to the same side.

Other angles in a rectangular trapezoid are always acute and obtuse. Moreover, their sum will always be equal to 180 degrees.

Each diagonal forms a right triangle with its smaller side. And the height, which is drawn from a vertex with an obtuse angle, divides the figure into two. One of them is a rectangle, and the other is a right triangle. By the way, this side is always equal to the height of the trapezoid.

What notations are used in the presented formulas?

It is convenient to immediately specify all quantities used in different expressions that describe a trapezoid and present them in a table:

Formulas that describe the elements of a rectangular trapezoid

The simplest of them relates height and smaller side:

A few more formulas for this side of a rectangular trapezoid:

с = d *sinα;

c = (a - b) * tan α;

c = √ (d 2 - (a - b) 2).

The first follows from a right triangle. And it says that the leg to the hypotenuse gives the sine of the opposite angle.

In the same triangle, the second leg is equal to the difference of the two bases. Therefore, the statement that equates the tangent of an angle to the ratio of the legs is true.

From the same triangle, a formula can be derived based on knowledge of the Pythagorean theorem. This is the third expression recorded.

You can write down formulas for the other side. There are also three of them:

d = (a - b) /cosα;

d = c / sin α;

d = √ (c 2 + (a - b) 2).

The first two are again obtained from the ratio of the sides in the same right triangle, and the second is derived from the Pythagorean theorem.

What formula can you use to calculate area?

The one given for the free trapezoid. You just need to take into account that the height is the side perpendicular to the bases.

S = (a + b) * h / 2.

These quantities are not always given explicitly. Therefore, to calculate the area of a rectangular trapezoid, you will need to perform some mathematical calculations.

What if you need to calculate diagonals?

In this case, you need to see that they form two right triangles. This means you can always use the Pythagorean theorem. Then the first diagonal will be expressed as follows:

d1 = √ (c 2 + b 2)

or in another way, replacing “c” with “h”:

d1 = √ (h 2 + b 2).

The formulas for the second diagonal are obtained in a similar way:

d2 = √ (c 2 + b 2) or d 2 = √ (h 2 + a 2).

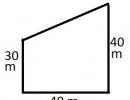
Task No. 1

Condition. The area of a rectangular trapezoid is known and equal to 120 dm 2. Its height has a length of 8 cm. It is necessary to calculate all sides of the trapezoid. An additional condition is that one base is 6 dm smaller than the other.

Solution. Since we are given a rectangular trapezoid in which the height is known, we can immediately say that one of the sides is 8 dm, that is, the smaller side.

Now you can count the other one: d = √ (c 2 + (a - b) 2). Moreover, here both the side c and the difference of the bases are given at once. The latter is equal to 6 dm, this is known from the condition. Then d will be equal to the square root of (64 + 36), that is, of 100. This is how another side is found, equal to 10 dm.

The sum of the bases can be found from the formula for area. It will be equal to twice the area divided by the height. If you count, it turns out 240 / 8. This means that the sum of the bases is 30 dm. On the other hand, their difference is 6 dm. By combining these equations, you can count both bases:

a + b = 30 and a - b = 6.

You can express a as (b + 6), substitute it into the first equality. Then it turns out that 2b will be equal to 24. Therefore, simply b will turn out to be 12 dm.

Then the last side a is 18 dm.

Answer. Sides of a rectangular trapezoid: a = 18 dm, b = 12 dm, c = 8 dm, d = 10 dm.

Task No. 2

Condition. Given a rectangular trapezoid. Its major side is equal to the sum of the bases. Its height is 12 cm long. A rectangle is constructed, the sides of which are equal to the bases of the trapezoid. It is necessary to calculate the area of this rectangle.

Solution. You need to start with what you are looking for. The required area is determined as the product of a and b. Both of these quantities are unknown.

It will be necessary to use additional equalities. One of them is based on the statement from the condition: d = a + b. It is necessary to use the third formula for this side, which is given above. It turns out: d 2 = c 2 + (a - b) 2 or (a + b) 2 = c 2 + (a - b) 2.

It is necessary to make transformations by substituting instead of c its value from the condition - 12. After opening the brackets and bringing similar terms, it turns out that 144 = 4 ab.

At the beginning of the solution it was said that a*b gives the required area. Therefore, in the last expression you can replace this product with S. A simple calculation will give the area value. S = 36 cm 2.

Answer. The required area is 36 cm 2.

Task No. 3

Condition. The area of a rectangular trapezoid is 150√3 cm². An acute angle is 60 degrees. The angle between the small base and the smaller diagonal has the same meaning. We need to calculate the smaller diagonal.

Solution. From the properties of the angles of a trapezoid, it turns out that its obtuse angle is 120º. Then the diagonal divides it into equal parts, because one part of it is already 60 degrees. Then the angle between this diagonal and the second base is also 60 degrees. That is, a triangle formed by a large base, an inclined side and a smaller diagonal is equilateral. Thus, the desired diagonal will be equal to a, as well as the side side d = a.

Now we need to consider a right triangle. The third angle in it is 30 degrees. This means that the leg opposite it is equal to half the hypotenuse. That is, the smaller base of the trapezoid is equal to half of the desired diagonal: b = a/2. From it you need to find the height equal to the side perpendicular to the bases. The side with the leg here. From the Pythagorean theorem:

c = (a/2) * √3.

Now all that remains is to substitute all the quantities into the area formula:

150√3 = (a + a/2) * (a/2 * √3) / 2.

Solving this equation gives the root 20

Answer. The smaller diagonal has a length of 20 cm.

Trapezoid is a quadrilateral that has two parallel sides, which are the bases, and two non-parallel sides, which are the sides.

There are also names such as isosceles or equilateral.

is a trapezoid whose side angles are right.

Trapezoid elements

a, b - trapezoid bases(a parallel to b),

m, n - sides trapezoids,

d 1 , d 2 — diagonals trapezoids,

h - height trapezoid (a segment connecting the bases and at the same time perpendicular to them),

MN - middle line(segment connecting the midpoints of the sides).

Area of trapezoid

Through the half-sum of bases a, b and height h: S = \frac(a + b)(2)\cdot h
Through the center line MN and height h: S = MN\cdot h
Through the diagonals d 1, d 2 and the angle (\sin \varphi) between them: S = \frac(d_(1) d_(2) \sin \varphi)(2)

Properties of a trapezoid

Midline of trapezoid

middle line parallel to the bases, equal to their half-sum and divides each segment with ends located on straight lines that contain the bases (for example, the height of the figure) in half:

MN || a, MN || b, MN = \frac(a + b)(2)

Sum of trapezoid angles

Sum of trapezoid angles, adjacent to each side, is equal to 180^(\circ) :

\alpha + \beta = 180^(\circ)

\gamma + \delta =180^(\circ)

Equal-area trapezoid triangles

Equal in size, that is, having equal areas, are the diagonal segments and triangles AOB and DOC formed by the lateral sides.

The similarity of the formed trapezoid triangles

Similar triangles are AOD and COB, which are formed by their bases and diagonal segments.

\triangle AOD \sim \triangle COB

Similarity coefficient k is found by the formula:

k = \frac(AD)(BC)

Moreover, the ratio of the areas of these triangles is equal to k^(2) .

Ratio of lengths of segments and bases

Each segment connecting the bases and passing through the point of intersection of the diagonals of the trapezoid is divided by this point in the ratio:

\frac(OX)(OY) = \frac(BC)(AD)

This will also be true for the height with the diagonals themselves.

A trapezoid is a flat four square, whose two opposite sides are parallel. They are called bases trapezoids, and the other two sides are the lateral sides trapezoids.

Instructions

The problem of finding an arbitrary angle in trapezoids requires a sufficient amount of additional data. Consider an example in which two base angles are known trapezoids. Let the angles &ang-BAD and &ang-CDA be known, let us find the angles &ang-ABC and &ang-BCD. A trapezoid has the property that the sum of the angles on each side is 180°. Then &ang-ABC = 180°--&ang-BAD, and &ang-BCD = 180°--&ang-CDA.

trapezoid" class="lightbx" data-lightbox="article-image">

Another problem may indicate equality of sides trapezoids and some additional angles. For example, as in the figure, it may be known that the sides AB, BC and CD are equal, and the diagonal makes an angle &ang-CAD = α- with the lower base. Consider three square ABC, it is isosceles, since AB = BC. Then &ang-BAC = &ang-BCA. Let's denote it x for brevity, and &ang-ABC - y. The sum of the angles of any three square a is equal to 180°-, it follows that 2x + y = 180°-, then y = 180°- - 2x. At the same time, from the properties trapezoids: y + x + α- = 180°- and therefore 180°- - 2x + x + α- = 180°-. Thus x = α-. We found two corners trapezoids: &ang-BAC = 2x = 2α- and &ang-ABC = y = 180°- - 2α-. Since AB = CD by condition, the trapezoid is isosceles or isosceles. Means,

Angles of an isosceles trapezoid. Hello! This article will focus on solving problems with trapezoids. This group of tasks is part of the exam; the problems are simple. We will calculate the angles of the trapezoid, base and height. Solving a number of problems comes down to solving, as they say: where are we without the Pythagorean theorem?

We will work with an isosceles trapezoid. It has equal sides and angles at the bases. There is an article on the trapezoid on the blog.

Let us note a small and important nuance, which we will not describe in detail in the process of solving the tasks themselves. Look, if we are given two bases, then the larger base with the heights lowered to it is divided into three segments - one is equal to the smaller base (these are the opposite sides of the rectangle), the other two are equal to each other (these are the legs of equal right triangles):

A simple example: given two bases of an isosceles trapezoid 25 and 65. The larger base is divided into segments as follows:

*And further! Letter symbols are not included in the problems. This was done deliberately so as not to overload the solution with algebraic refinements. I agree that this is mathematically illiterate, but the goal is to get the point across. And you can always make the designations for vertices and other elements yourself and write down a mathematically correct solution.

Let's consider the tasks:

27439. The bases of an isosceles trapezoid are 51 and 65. The sides are 25. Find the sine of the acute angle of the trapezoid.

In order to find the angle, you need to construct the heights. In the sketch we denote the data in the quantity condition. The lower base is 65, with heights it is divided into segments 7, 51 and 7:

In a right triangle, we know the hypotenuse and leg, we can find the second leg (the height of the trapezoid) and then calculate the sine of the angle.

According to the Pythagorean theorem, the indicated leg is equal to:

Thus:

Answer: 0.96

27440. The bases of an isosceles trapezoid are 43 and 73. The cosine of an acute angle of a trapezoid is 5/7. Find the side.

Let's construct the heights and note the data in the magnitude condition; the lower base is divided into segments 15, 43 and 15:

27441. The greater base of an isosceles trapezoid is 34. The side is 14. The sine of an acute angle is (2√10)/7. Find the smaller base.

Let's build heights. In order to find the smaller base, we need to find what the segment that is the leg in the right triangle is equal to (indicated in blue):

We can calculate the height of the trapezoid and then find the leg:

Using the Pythagorean theorem we calculate the leg:

So the smaller base is:

27442. The bases of an isosceles trapezoid are 7 and 51. The tangent of an acute angle is 5/11. Find the height of the trapezoid.

Let's construct the heights and mark the data in the magnitude condition. The lower base is divided into segments:

What to do? We express the tangent of the angle we know at the base in a right triangle:

27443. The smaller base of an isosceles trapezoid is 23. The height of the trapezoid is 39. The tangent of an acute angle is 13/8. Find a larger base.

We build the heights and calculate what the leg is equal to:

Thus the larger base will be equal to:

27444. The bases of an isosceles trapezoid are 17 and 87. The height of the trapezoid is 14. Find the tangent of the acute angle.

We build heights and mark known values on the sketch. The lower base is divided into segments 35, 17, 35:

By definition of tangent:

77152. The bases of an isosceles trapezoid are 6 and 12. The sine of an acute angle of a trapezoid is 0.8. Find the side.

Let's build a sketch, construct heights and mark known values, the larger base is divided into segments 3, 6 and 3:

Let's express the hypotenuse, designated as x, through the cosine:

From the main trigonometric identity we find cosα

Thus:

27818. What is the greater angle of an isosceles trapezoid if it is known that the difference between the opposite angles is 50 0? Give your answer in degrees.

From the geometry course we know that if we have two parallel lines and a transversal, the sum of the internal one-sided angles is equal to 180 0. In our case it is