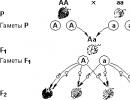
Equations with a parameter. Quadratic equations with parameters Logarithmic equations with parameter
Equation of the form f(x; a) = 0 is called equation with variable X and parameter A.
Solve equation with parameter A– this means for each value A find values X, satisfying this equation.
Example 1. Oh= 0
Example 2. Oh = A
Example 3.
x + 2 = ah
x – ah = -2
x(1 – a) = -2
If 1 – A= 0, i.e. A= 1, then X 0 = -2 no roots
If 1 – A 0, i.e. A 1, then X =
Example 4.
(A 2 – 1) X = 2A 2 + A – 3
(A – 1)(A + 1)X = 2(A – 1)(A – 1,5)
(A – 1)(A + 1)X = (1A – 3)(A – 1)
If A= 1, then 0 X = 0
X– any real number
If A= -1, then 0 X = -2
no roots
If A 1, A-1, then X= (the only solution).
This means that for each valid value A matches a single value X.
For example:
If A= 5, then X = = ;
If A= 0, then X= 3, etc.
Didactic material
1. Oh = X + 3
2. 4 + Oh = 3X – 1
3. A = +
at A= 1 no roots.
at A= 3 no roots.
at A = 1 X– any real number except X = 1
at A = -1, A= 0 no solutions.
at A = 0, A= 2 no solutions.
at A = -3, A = 0, 5, A= -2 no solutions
at A = -With, With= 0 no solutions.
Quadratic equations with parameter
Example 1. Solve the equation
(A – 1)X 2 = 2(2A + 1)X + 4A + 3 = 0
At A = 1 6X + 7 = 0
When A 1, we highlight those parameter values at which D goes to zero.
D = (2(2 A + 1)) 2 – 4(A – 1)(4A + 30 = 16A 2 + 16A + 4 – 4(4A 2 + 3A – 4A – 3) = 16A 2 + 16A + 4 – 16A 2 + 4A + 12 = 20A + 16
20A + 16 = 0
20A = -16
If A < -4/5, то D < 0, уравнение имеет действительный корень.
If A> -4/5 and A 1, then D > 0,
X = ![]()
If A= 4/5, then D = 0,
Example 2. At what values of the parameter a does the equation
x 2 + 2( A + 1)X + 9A– 5 = 0 has 2 different negative roots?
D = 4( A + 1) 2 – 4(9A – 5) = 4A 2 – 28A + 24 = 4(A – 1)(A – 6)
4(A – 1)(A – 6) > 0
via t. Vieta: X 1 + X 2 = -2(A + 1)
X 1 X 2 = 9A – 5
By condition X 1 < 0, X 2 < 0 то –2(A + 1) < 0 и 9A – 5 > 0
| Eventually | 4(A – 1)(A – 6) > 0 - 2(A + 1) < 0 9A – 5 > 0 |
A < 1: а > 6 A > - 1 A > 5/9 |
(Rice. 1) < a < 1, либо a > 6 |
Example 3. Find the values A, at which given equation has a solution.
x 2 – 2( A – 1)X + 2A + 1 = 0
D = 4( A – 1) 2 – 4(2A + 10 = 4A 2 – 8A + 4 – 8A – 4 = 4A 2 – 16A
4A 2 – 16 0
4A(A – 4) 0
A( A – 4)) 0
A( A – 4) = 0
a = 0 or A – 4 = 0
A = 4
(Rice. 2)
Answer: A 0 and A 4
Didactic material
1. At what value A the equation Oh 2 – (A + 1) X + 2A– 1 = 0 has one root?
2. At what value A the equation ( A + 2) X 2 + 2(A + 2)X+ 2 = 0 has one root?
3. For what values of a is the equation ( A 2 – 6A + 8) X 2 + (A 2 – 4) X + (10 – 3A – A 2) = 0 has more than two roots?
4. For what values of a, equation 2 X 2 + X – A= 0 has at least one common root with equation 2 X 2 – 7X + 6 = 0?
5. For what values of a the equation X 2 +Oh+ 1 = 0 and X 2 + X + A= 0 have at least one common root?
1. When A = - 1/7, A = 0, A = 1
2. When A = 0
3. When A = 2
4. When A = 10
5. When A = - 2
Exponential equations with parameter
Example 1.Find all values A, for which the equation
9 x – ( A+ 2)*3 x-1/x +2 A*3 -2/x = 0 (1) has exactly two roots.
Solution. Multiplying both sides of equation (1) by 3 2/x, we obtain the equivalent equation
3 2(x+1/x) – ( A+ 2)*3 x+1/x + 2 A = 0 (2)
Let 3 x+1/x = at, then equation (2) will take the form at 2 – (A + 2)at + 2A= 0, or
(at – 2)(at – A) = 0, whence at 1 =2, at 2 = A.
If at= 2, i.e. 3 x+1/x = 2 then X + 1/X= log 3 2 , or X 2 – X log 3 2 + 1 = 0.
This equation has no real roots, since it D= log 2 3 2 – 4< 0.
If at = A, i.e. 3 x+1/x = A That X + 1/X= log 3 A, or X 2 –X log 3 a + 1 = 0. (3)
Equation (3) has exactly two roots if and only if
D = log 2 3 2 – 4 > 0, or |log 3 a| > 2.
If log 3 a > 2, then A> 9, and if log 3 a< -2, то 0 < A < 1/9.
Answer: 0< A < 1/9, A > 9.
Example 2. At what values of a is the equation 2 2х – ( A - 3) 2 x – 3 A= 0 has solutions?
In order for a given equation to have solutions, it is necessary and sufficient that the equation t 2 – (a – 3) t – 3a= 0 had at least one positive root. Let's find the roots using Vieta's theorem: X 1 = -3, X 2 = A = >
a is a positive number.
Answer: when A > 0
Didactic material
1. Find all values of a for which the equation
25 x – (2 A+ 5)*5 x-1/x + 10 A* 5 -2/x = 0 has exactly 2 solutions.
2. For what values of a is the equation
2 (a-1)x?+2(a+3)x+a = 1/4 has a single root?
3. At what values of the parameter a does the equation
4 x - (5 A-3)2 x +4 A 2 – 3A= 0 has a unique solution?
Logarithmic equations with parameter
Example 1. Find all values A, for which the equation
log 4x (1 + Oh) = 1/2 (1)
has a unique solution.
Solution. Equation (1) is equivalent to equation
1 + Oh = 2X at X > 0, X 1/4 (3)
X = at
ay 2 – at + 1 = 0 (4)
Condition (2) from (3) is not satisfied.
Let A 0, then AU 2 – 2at+ 1 = 0 has real roots if and only if D = 4 – 4A 0, i.e. at A 1.To solve inequality (3), let’s plot the functions Galitsky M.L., Moshkovich M.M., Shvartsburd S.I. In-depth study of the course of algebra and mathematical analysis. – M.: Education, 1990
1. Task.
At what parameter values a the equation ( a - 1)x 2 + 2x + a- Does 1 = 0 have exactly one root?
1. Solution.
At a= 1 the equation is 2 x= 0 and obviously has a single root x= 0. If a No. 1, then this equation is quadratic and has a single root for those parameter values at which the discriminant quadratic trinomial equal to zero. Equating the discriminant to zero, we obtain an equation for the parameter a
4a 2 - 8a= 0, whence a= 0 or a = 2.
1. Answer: the equation has a single root at a O (0; 1; 2).
2. Task.
Find all parameter values a, for which the equation has two different roots x 2 +4ax+8a+3 = 0.
2. Solution.
The equation x 2 +4ax+8a+3 = 0 has two distinct roots if and only if D =
16a 2 -4(8a+3) > 0. We get (after reduction by a common factor of 4) 4 a 2 -8a-3 > 0, whence
2. Answer:
| a O (-Ґ ; 1 – | Ts 7 2 |
) AND (1 + | Ts 7 2 |
; Ґ ). |
3. Task.
It is known that
f 2 (x) = 6x-x 2 -6.
a) Graph the function f 1 (x) at a = 1.
b) At what value a function graphs f 1 (x) And f 2 (x) have a single common point?
3. Solution.
3.a. Let's transform f 1 (x) in the following way
The graph of this function at a= 1 is shown in the figure on the right.
3.b. Let us immediately note that the graphs of functions y =
kx+b And y = ax 2 +bx+c
(a No. 0) intersect at a single point if and only if the quadratic equation kx+b =
ax 2 +bx+c has a single root. Using View f 1 of 3.a, let us equate the discriminant of the equation a = 6x-x 2 -6 to zero. From equation 36-24-4 a= 0 we get a= 3. Do the same with equation 2 x-a = 6x-x 2 -6 we will find a= 2. It is easy to verify that these parameter values satisfy the conditions of the problem. Answer: a= 2 or a = 3.
4. Task.
Find all values a, for which the set of solutions to the inequality x 2 -2ax-3a i 0 contains the segment .
4. Solution.
First coordinate of the parabola vertex f(x) =
x 2 -2ax-3a equal to x 0 =
a. From properties quadratic function condition f(x) і 0 on the segment is equivalent to a set of three systems
has exactly two solutions?
5. Solution.
Let us rewrite this equation in the form x 2 + (2a-2)x - 3a+7 = 0. This is a quadratic equation; it has exactly two solutions if its discriminant is strictly greater than zero. Calculating the discriminant, we find that the condition for the presence of exactly two roots is the fulfillment of the inequality a 2 +a-6 > 0. Solving the inequality, we find a < -3 или a> 2. The first of the inequalities, obviously, has no solutions in natural numbers, and the smallest natural solution to the second is the number 3.
5. Answer: 3.
6. Problem (10 keys)
Find all values a, for which the graph of the function or, after obvious transformations, a-2 = |
2-a| . The last equation is equivalent to the inequality a i 2.
6. Answer: a ABOUT )