What does a fraction look like in decimal notation. Decimals. The concept of decimal fraction. Convert decimal to ordinary
We have already said that fractions are ordinary And decimal. On the this moment We learned a little about common fractions. We learned that there are regular fractions and improper fractions. We also learned that ordinary fractions can be reduced, added, subtracted, multiplied and divided. And we also learned that there are so-called mixed numbers, which consist of an integer and a fractional part.
We have not yet fully studied ordinary fractions. There are many subtleties and details that should be discussed, but today we will begin to study decimal fractions, because ordinary and decimals quite often have to be combined. That is, when solving problems, you have to work with both types of fractions.
This lesson may seem complicated and incomprehensible. It's quite normal. These kinds of lessons require that they be studied and not skimmed over.
Lesson contentExpressing quantities in fractional form
Sometimes it is convenient to show something in fractional form. For example, one tenth of a decimeter is written like this:
This expression means that one decimeter was divided into ten equal parts, and one part was taken from these ten parts. And one part out of ten this case is equal to one centimeter:

Consider the following example. Show 6 cm and another 3 mm in centimeters in fractional form.
So, you want to show 6 cm and 3 mm in centimeters, but in fractional form. We already have 6 whole centimeters:

But there are still 3 millimeters left. How to show these 3 millimeters, while in centimeters? Fractions come to the rescue. One centimeter is ten millimeters. Three millimeters is three parts out of ten. And three parts out of ten are written as cm

The expression cm means that one centimeter was divided into ten equal parts, and three parts were taken from these ten parts.
As a result, we have six whole centimeters and three tenths of a centimeter:
In this case, 6 shows the number of whole centimeters, and the fraction shows the number of fractional. This fraction is read as "six point and three tenths of a centimeter".
Fractions, in the denominator of which there are numbers 10, 100, 1000, can be written without a denominator. First write the integer part, and then the numerator of the fractional part. The integer part is separated from the numerator of the fractional part by a comma.
For example, let's write without a denominator. First write down the whole part. The whole part is 6
The whole part is recorded. Immediately after writing the whole part, put a comma:
And now we write down the numerator of the fractional part. In a mixed number, the numerator of the fractional part is the number 3. We write the three after the decimal point:
Any number that is represented in this form is called decimal.
Therefore, you can show 6 cm and another 3 mm in centimeters using a decimal fraction:
6.3 cm
It will look like this:

In fact, decimals are the same common fractions and mixed numbers. The peculiarity of such fractions is that the denominator of their fractional part contains the numbers 10, 100, 1000 or 10000.
Like a mixed number, a decimal has an integer part and a fractional part. For example, in a mixed number, the integer part is 6 and the fractional part is .
In the decimal fraction 6.3, the integer part is the number 6, and the fractional part is the numerator of the fraction, that is, the number 3.
It also happens that ordinary fractions in the denominator of which the numbers 10, 100, 1000 are given without an integer part. For example, a fraction is given without an integer part. To write such a fraction as a decimal, first write down 0, then put a comma and write down the numerator of the fractional part. A fraction without a denominator would be written like this:
Reads like "zero point five tenths".
Convert mixed numbers to decimals
When we write mixed numbers without a denominator, we are converting them to decimals. When converting ordinary fractions to decimal fractions, there are a few things you need to know, which we'll talk about now.
After the integer part is written, it is imperative to count the number of zeros in the denominator of the fractional part, since the number of zeros in the fractional part and the number of digits after the decimal point in the decimal fraction must be the same. What does it mean? Consider the following example:
At first
And you could immediately write down the numerator of the fractional part and the decimal fraction is ready, but you must definitely count the number of zeros in the denominator of the fractional part.
So, we count the number of zeros in the fractional part of the mixed number. The denominator of the fractional part has one zero. So in the decimal fraction after the decimal point there will be one digit and this figure will be the numerator of the fractional part of the mixed number, that is, the number 2
Thus, the mixed number, when translated into a decimal fraction, becomes 3.2.
This decimal is read like this:
"Three whole two tenths"
"Tenths" because the fractional part of the mixed number contains the number 10.
Example 2 Convert mixed number to decimal.
We write down the whole part and put a comma:
And you could immediately write down the numerator of the fractional part and get the decimal fraction 5.3, but the rule says that after the decimal point there should be as many digits as there are zeros in the denominator of the fractional part of the mixed number. And we see that there are two zeros in the denominator of the fractional part. So in our decimal fraction after the decimal point there should be two digits, not one.
In such cases, the numerator of the fractional part needs to be slightly modified: add a zero before the numerator, that is, before the number 3
Now you can convert this mixed number to a decimal. We write down the whole part and put a comma:
And write the numerator of the fractional part:
The decimal fraction 5.03 reads like this:
"Five point three hundredths"
"Hundredths" because the denominator of the fractional part of the mixed number is the number 100.
Example 3 Convert mixed number to decimal.
From the previous examples, we learned that in order to successfully convert a mixed number to a decimal, the number of digits in the numerator of the fractional part and the number of zeros in the denominator of the fractional part must be the same.
Before converting a mixed number into a decimal fraction, its fractional part needs to be slightly modified, namely, to make sure that the number of digits in the numerator of the fractional part and the number of zeros in the denominator of the fractional part are the same.
First of all, we look at the number of zeros in the denominator of the fractional part. We see that there are three zeros:

Our task is to organize three digits in the numerator of the fractional part. We already have one digit - this is the number 2. It remains to add two more digits. They will be two zeros. Add them before the number 2. As a result, the number of zeros in the denominator and the number of digits in the numerator will become the same:

Now we can turn this mixed number into a decimal. We write down the whole part first and put a comma:
and immediately write down the numerator of the fractional part
3,002
We see that the number of digits after the decimal point and the number of zeros in the denominator of the fractional part of the mixed number are the same.
The decimal 3.002 reads like this:
"Three whole, two thousandths"
"Thousandths" because the denominator of the fractional part of the mixed number is the number 1000.
Converting common fractions to decimals
Ordinary fractions, in which the denominator is 10, 100, 1000 or 10000, can also be converted to decimal fractions. Since an ordinary fraction does not have an integer part, first write down 0, then put a comma and write down the numerator of the fractional part.
Here, too, the number of zeros in the denominator and the number of digits in the numerator must be the same. Therefore, you should be careful.
Example 1
The integer part is missing, so first we write 0 and put a comma:
Now look at the number of zeros in the denominator. We see that there is one zero. And the numerator has one digit. So you can safely continue the decimal fraction by writing the number 5 after the decimal point
In the resulting decimal fraction 0.5, the number of digits after the decimal point and the number of zeros in the denominator of the fraction are the same. So the fraction is correct.
The decimal fraction 0.5 reads like this:
"Zero point, five tenths"
Example 2 Convert common fraction to decimal.
The whole part is missing. We write 0 first and put a comma:
Now look at the number of zeros in the denominator. We see that there are two zeros. And the numerator has only one digit. To make the number of digits and the number of zeros the same, add one zero in the numerator before the number 2. Then the fraction will take the form . Now the number of zeros in the denominator and the number of digits in the numerator are the same. So you can continue the decimal:
In the resulting decimal fraction 0.02, the number of digits after the decimal point and the number of zeros in the denominator of the fraction are the same. So the fraction is correct.
The decimal fraction 0.02 reads like this:
"Zero point, two hundredths."
Example 3 Convert common fraction to decimal.
We write 0 and put a comma:
Now we count the number of zeros in the denominator of the fraction. We see that there are five zeros, and there is only one digit in the numerator. To make the number of zeros in the denominator and the number of digits in the numerator the same, you need to add four zeros in the numerator before the number 5:
Now the number of zeros in the denominator and the number of digits in the numerator are the same. So you can continue the decimal. We write down the numerator of the fraction after the decimal point
In the resulting decimal fraction 0.00005, the number of digits after the decimal point and the number of zeros in the denominator of the fraction are the same. So the fraction is correct.
The decimal fraction 0.00005 reads like this:
"Zero point, five hundred-thousandths."
Convert improper fractions to decimals
An improper fraction is a fraction whose numerator is greater than the denominator. There are improper fractions that have the numbers 10, 100, 1000 or 10000 in the denominator. Such fractions can be converted to decimal fractions. But before converting to a decimal fraction, such fractions must have an integer part.
Example 1
The fraction is an improper fraction. To convert such a fraction to a decimal fraction, you must first select its integer part. We recall how to select the whole part of improper fractions. If you forgot, we advise you to return to and study it.
So, let's select the integer part in the improper fraction. Recall that a fraction means division - in this case, dividing the number 112 by the number 10

Let's look at this picture and assemble a new mixed number, like a children's construction set. The number 11 will be the integer part, the number 2 will be the numerator of the fractional part, the number 10 will be the denominator of the fractional part.

We got a mixed number. Let's convert it to a decimal. And we already know how to translate such numbers into decimal fractions. First we write down the whole part and put a comma:
Now we count the number of zeros in the denominator of the fractional part. We see that there is one zero. And the numerator of the fractional part has one digit. This means that the number of zeros in the denominator of the fractional part and the number of digits in the numerator of the fractional part are the same. This gives us the opportunity to immediately write the numerator of the fractional part after the decimal point:
In the resulting decimal fraction 11.2, the number of digits after the decimal point and the number of zeros in the denominator of the fraction are the same. So the fraction is correct.
This means that an improper fraction, when converted to a decimal fraction, turns into 11.2
Decimal 11.2 reads like this:
"Eleven whole, two tenths."
Example 2 Convert improper fraction to decimal.
This is an improper fraction because the numerator is greater than the denominator. But it can be converted to a decimal fraction, since the denominator is the number 100.
First of all, we select the integer part of this fraction. To do this, divide 450 by 100 by a corner:

Let's collect a new mixed number - we get . And we already know how to translate mixed numbers into decimal fractions.
We write down the whole part and put a comma:
Now we count the number of zeros in the denominator of the fractional part and the number of digits in the numerator of the fractional part. We see that the number of zeros in the denominator and the number of digits in the numerator are the same. This gives us the opportunity to immediately write the numerator of the fractional part after the decimal point:
In the resulting decimal fraction 4.50, the number of digits after the decimal point and the number of zeros in the denominator of the fraction are the same. So the fraction is translated correctly.
So the improper fraction, when translated into a decimal fraction, turns into 4.50
When solving problems, if there are zeros at the end of the decimal fraction, they can be discarded. Let's drop the zero in our answer. Then we get 4.5
This is one of the interesting features of decimals. It lies in the fact that the zeros that are at the end of the fraction do not give this fraction any weight. In other words, the decimals 4.50 and 4.5 are equal. Let's put an equal sign between them:
4,50 = 4,5
The question arises: why is this happening? After all, 4.50 and 4.5 look like different fractions. The whole secret lies in the basic property of the fraction, which we studied earlier. We will try to prove why the decimal fractions 4.50 and 4.5 are equal, but after studying the next topic, which is called "converting a decimal fraction to a mixed number."
Decimal to mixed number conversion
Any decimal fraction can be converted back to a mixed number. To do this, it is enough to be able to read decimal fractions. For example, let's convert 6.3 to a mixed number. 6.3 is six whole points and three tenths. We write down six integers first:
and next three tenths:
Example 2 Convert decimal 3.002 to mixed number
3.002 is three integers and two thousandths. Write down three integers first.
and next we write two thousandths:
Example 3 Convert decimal 4.50 to mixed number
4.50 is four point and fifty hundredths. Write down four integers
and next fifty hundredths:
By the way, let's remember the last example from the previous topic. We said that the decimals 4.50 and 4.5 are equal. We also said that zero can be discarded. Let's try to prove that decimal 4.50 and 4.5 are equal. To do this, we convert both decimal fractions to mixed numbers.
After converting to a mixed number, the decimal 4.50 becomes , and the decimal 4.5 becomes
We have two mixed numbers and . Convert these mixed numbers to improper fractions:
![]()
![]()
Now we have two fractions and . It is time to remember the basic property of a fraction, which says that when multiplying (or dividing) the numerator and denominator of a fraction by the same number, the value of the fraction does not change.
Let's divide the first fraction by 10
![]()
Received, and this is the second fraction. So and are equal to each other and equal to the same value:
Try dividing 450 by 100 first on a calculator, and then 45 by 10. A funny thing will work out.
Convert decimal to common fraction
Any decimal fraction can be converted back to a common fraction. To do this, again, it is enough to be able to read decimal fractions. For example, let's convert 0.3 to an ordinary fraction. 0.3 is zero and three tenths. We write zero integers first:
and next to three tenths 0 . Zero is traditionally not written down, so the final answer will not be 0, but simply.
Example 2 Convert decimal 0.02 to common fraction.
0.02 is zero and two hundredths. We don’t write down zero, so we immediately write down two hundredths
Example 3 Convert 0.00005 to fraction
0.00005 is zero and five hundred thousandths. Zero is not written down, so we immediately write down five hundred thousandths
Did you like the lesson?
Join our new Vkontakte group and start receiving notifications of new lessons
We will devote this material to such important topic like decimals. First, let's define the basic definitions, give examples and dwell on the rules of decimal notation, as well as what the digits of decimal fractions are. Next, we highlight the main types: finite and infinite, periodic and non-periodic fractions. In the final part, we will show how the points corresponding to fractional numbers are located on the coordinate axis.
What is decimal notation for fractional numbers
The so-called decimal notation for fractional numbers can be used for both natural and fractional numbers. It looks like a set of two or more numbers with a comma between them.
The decimal point is used to separate the integer part from the fractional part. As a rule, the last digit of a decimal is never a zero, unless the decimal point is immediately after the first zero.
What are some examples of fractional numbers in decimal notation? It can be 34 , 21 , 0 , 35035044 , 0 , 0001 , 11 231 552 , 9 etc.
In some textbooks, you can find the use of a dot instead of a comma (5. 67, 6789. 1011, etc.). This option is considered equivalent, but it is more typical for English-language sources.
Definition of decimals
Based on the above concept of decimal notation, we can formulate the following definition of decimal fractions:
Definition 1
Decimals represent fractional numbers in decimal notation.
Why do we need to write fractions in this form? It gives us some advantages over ordinary ones, for example, a more compact notation, especially in cases where the denominator is 1000, 100, 10, etc. or a mixed number. For example, instead of 6 10 we can specify 0 , 6 , instead of 25 10000 - 0 , 0023 , instead of 512 3 100 - 512 , 03 .
How to correctly represent ordinary fractions with tens, hundreds, thousands in the denominator in decimal form will be described in a separate material.
How to read decimals correctly
There are some rules for reading records of decimals. So, those decimal fractions that correspond to their correct ordinary equivalents are read almost the same, but with the addition of the words "zero tenths" at the beginning. So, the entry 0 , 14 , which corresponds to 14 100 , is read as "zero point fourteen hundredths."
If a decimal fraction can be associated with a mixed number, then it is read in the same way as this number. So, if we have a fraction 56, 002, which corresponds to 56 2 1000, we read such an entry as "fifty-six point two thousandths."
The value of a digit in a decimal notation depends on where it is located (just like in the case of natural numbers). So, in decimal fraction 0, 7, seven is tenths, in 0, 0007 it is ten thousandths, and in fraction 70,000, 345 it means seven tens of thousands of whole units. Thus, in decimal fractions, there is also the concept of a number digit.
The names of the digits located before the comma are similar to those that exist in natural numbers. The names of those that are located after are clearly presented in the table:
Let's take an example.
Example 1
We have decimal 43, 098. She has a four in the tens place, a three in the units place, zero in the tenth place, 9 in the hundredth place, and 8 in the thousandth place.
It is customary to distinguish the digits of decimal fractions by seniority. If we move through the numbers from left to right, then we will go from high to low digits. It turns out that hundreds are older than tens, and millionths are younger than hundredths. If we take that final decimal fraction, which we cited as an example above, then in it the senior, or highest, will be the digit of hundreds, and the lowest, or lowest, will be the digit of 10 thousandths.
Any decimal fraction can be decomposed into separate digits, that is, represented as a sum. This operation is performed in the same way as for natural numbers.
Example 2
Let's try to expand the fraction 56, 0455 into digits.
We will be able to:
56 , 0455 = 50 + 6 + 0 , 4 + 0 , 005 + 0 , 0005
If we remember the properties of addition, we can represent this fraction in other forms, for example, as the sum 56 + 0, 0455, or 56, 0055 + 0, 4, etc.
What are trailing decimals
All the fractions we talked about above are trailing decimals. This means that the number of digits after the decimal point is finite. Let's get the definition:
Definition 1
Trailing decimals are a type of decimal that has a finite number of digits after the comma.
Examples of such fractions can be 0, 367, 3, 7, 55, 102567958, 231032, 49, etc.
Any of these fractions can be converted either into a mixed number (if the value of their fractional part is different from zero), or into an ordinary fraction (if the integer part is zero). We have devoted a separate material to how this is done. Let's just point out a couple of examples here: for example, we can bring the final decimal fraction 5 , 63 to the form 5 63 100 , and 0 , 2 corresponds to 2 10 (or any other fraction equal to it, for example, 4 20 or 1 5 .)
But the reverse process, i.e. writing an ordinary fraction in decimal form may not always be performed. So, 5 13 cannot be replaced by an equal fraction with a denominator of 100, 10, etc., which means that the final decimal fraction will not work out of it.
The main types of infinite decimal fractions: periodic and non-periodic fractions
We pointed out above that finite fractions are called so because they have a finite number of digits after the decimal point. However, it may well be infinite, in which case the fractions themselves will also be called infinite.
Definition 2
Infinite decimals are those that have an infinite number of digits after the decimal point.
Obviously, such numbers simply cannot be written completely, so we indicate only a part of them and then put ellipsis. This sign indicates an infinite continuation of the sequence of decimal places. Examples of infinite decimals would be 0 , 143346732 ... , 3 , 1415989032 ... , 153 , 0245005 ... , 2 , 66666666666 ... , 69 , 748768152 ... . etc.
In the “tail” of such a fraction, there can be not only seemingly random sequences of numbers, but a constant repetition of the same character or group of characters. Fractions with alternation after the decimal point are called periodic.
Definition 3
Periodic decimal fractions are such infinite decimal fractions in which one digit or a group of several digits is repeated after the decimal point. The repeating part is called the period of the fraction.
For example, for the fraction 3, 444444 ... . the period will be the number 4, and for 76, 134134134134 ... - the group 134.
What minimal amount Is it permissible to leave signs in the record of a periodic fraction? For periodic fractions, it will be sufficient to write the entire period once in parentheses. So, the fraction is 3, 444444 ... . it will be correct to write as 3, (4) , and 76, 134134134134 ... - as 76, (134) .
In general, entries with multiple periods in brackets will have exactly the same meaning: for example, the periodic fraction 0.677777 is the same as 0.6 (7) and 0.6 (77), etc. Entries like 0 , 67777 (7) , 0 , 67 (7777) and others are also allowed.
In order to avoid errors, we introduce the uniformity of notation. Let's agree to write only one period (the shortest possible sequence of digits), which is closest to the decimal point, and enclose it in parentheses.
That is, for the above fraction, we will consider the entry 0, 6 (7) as the main one, and, for example, in the case of the fraction 8, 9134343434, we will write 8, 91 (34) .
If the denominator of an ordinary fraction contains prime factors that are not equal to 5 and 2, then when converted to decimal notation, infinite fractions will be obtained from them.
In principle, we can write any finite fraction as a periodic one. To do this, we just need to add an infinite number of zeros to the right. How does it look on the record? Let's say we have a final fraction 45, 32. In periodic form, it will look like 45 , 32 (0) . This action is possible because adding zeros to the right of any decimal fraction gives us a fraction equal to it as a result.
Separately, one should dwell on periodic fractions with a period of 9, for example, 4, 89 (9), 31, 6 (9) . They are an alternative notation for similar fractions with a period of 0, so they are often replaced when writing with fractions with a zero period. At the same time, one is added to the value of the next digit, and (0) is indicated in parentheses. The equality of the resulting numbers is easy to check by presenting them as ordinary fractions.
For example, the fraction 8, 31 (9) can be replaced by the corresponding fraction 8, 32 (0) . Or 4 , (9) = 5 , (0) = 5 .
Infinite decimal periodic fractions are rational numbers. In other words, any periodic fraction can be represented as an ordinary fraction, and vice versa.
There are also fractions in which there is no infinitely repeating sequence after the decimal point. In this case, they are called non-periodic fractions.
Definition 4
Non-periodic decimal fractions include those infinite decimal fractions that do not contain a period after the decimal point, i.e. repeating group of numbers.
Sometimes non-periodic fractions look very similar to periodic ones. For example, 9 , 03003000300003 ... at first glance it seems to have a period, however detailed analysis decimal places confirms that this is still a non-periodic fraction. You have to be very careful with numbers like this.
Non-periodic fractions are irrational numbers. They are not converted to ordinary fractions.
Basic operations with decimals
The following operations can be performed with decimal fractions: comparison, subtraction, addition, division and multiplication. Let's analyze each of them separately.
Comparing decimals can be reduced to comparing ordinary fractions that correspond to the original decimals. But infinite non-periodic fractions cannot be reduced to this form, and converting decimal fractions to ordinary ones is often a laborious task. How to quickly perform a comparison action if we need to do it in the course of solving the problem? It is convenient to compare decimal fractions by digits in the same way as we compare natural numbers. We will devote a separate article to this method.
To add one decimal fraction to another, it is convenient to use the column addition method, as for natural numbers. To add periodic decimal fractions, you must first replace them with ordinary ones and count according to the standard scheme. If, according to the conditions of the problem, we need to add infinite non-periodic fractions, then we must first round them up to a certain digit, and then add them. The smaller the digit to which we round, the higher the accuracy of the calculation will be. For subtraction, multiplication and division of infinite fractions, preliminary rounding is also necessary.
Finding the difference of decimal fractions is the opposite of addition. In fact, with the help of subtraction, we can find a number whose sum with the subtracted fraction will give us the reduced one. We will talk about this in more detail in a separate article.
Multiplication of decimal fractions is done in the same way as for natural numbers. The method of calculation by a column is also suitable for this. We again reduce this action with periodic fractions to the multiplication of ordinary fractions according to the rules already studied. Infinite fractions, as we remember, must be rounded before counting.
The process of dividing decimals is the reverse of the multiplication process. When solving problems, we also use column counts.
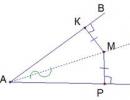
You can set an exact correspondence between the end decimal and a point on the coordinate axis. Let's figure out how to mark a point on the axis that will exactly correspond to the required decimal fraction.
We have already studied how to construct points corresponding to ordinary fractions, and decimal fractions can be reduced to this form. For example, the common fraction 14 10 is the same as 1 , 4 , so the point corresponding to it will be exactly the same distance from the origin in the positive direction:

You can do without replacing the decimal fraction with an ordinary one, and take the digit expansion method as a basis. So, if we need to mark a point whose coordinate will be equal to 15 , 4008 , then we will first represent this number as a sum 15 + 0 , 4 + , 0008 . To begin with, we set aside 15 whole unit segments in the positive direction from the origin, then 4 tenths of one segment, and then 8 ten-thousandths of one segment. As a result, we will get a coordinate point, which corresponds to the fraction 15, 4008.
For an infinite decimal fraction, it is better to use this particular method, since it allows you to approach the desired point as close as you like. In some cases, it is possible to build an exact correspondence of an infinite fraction on the coordinate axis: for example, 2 = 1, 41421. . . , and this fraction can be associated with a point on the coordinate ray, remote from 0 by the length of the diagonal of the square, the side of which will be equal to one unit segment.
If we find not a point on the axis, but a decimal fraction corresponding to it, then this action is called the decimal measurement of the segment. Let's see how to do it right.
Suppose we need to get from zero to a given point on the coordinate axis (or get as close as possible in the case of an infinite fraction). To do this, we gradually set aside unit segments from the origin of coordinates until we get to the desired point. After whole segments, if necessary, we measure tenths, hundredths and smaller parts so that the correspondence is as accurate as possible. As a result, we got a decimal fraction, which corresponds to given point on the coordinate axis.
Above we gave a picture with a point M. Look at it again: to get to this point, you need to measure one unit segment from zero and four tenths of it, since this point corresponds to the decimal fraction 1, 4.
If we cannot hit a point in the process of decimal measurement, then it means that an infinite decimal fraction corresponds to it.
If you notice a mistake in the text, please highlight it and press Ctrl+Enter
There is another view rational number 1/2, other than representations like 2/4, 3/6, 4/8, etc. We mean decimal representation 0.5. Some fractions have finite decimal representations, for example,
while the decimal representations of other fractions are infinite:

These infinite decimals can be obtained from the corresponding rational fractions by dividing the numerator by the denominator. For example, in the case of the fraction 5/11, dividing 5.000... by 11 gives 0.454545...
What rational fractions have finite decimal representations? Before answering this question in the general case, let's consider a specific example. Take, say, the final decimal fraction 0.8625. We know that
![]()
and that any finite decimal can be written as a rational decimal with a denominator equal to 10, 100, 1000, or some other power of 10.
Reducing the fraction on the right to an irreducible fraction, we get
![]()
The denominator 80 is obtained by dividing 10,000 by 125 - the largest common divisor 10,000 and 8625. Therefore, the prime factorization of the number 80, like the number 10,000, includes only two prime factors: 2 and 5. If we started not with 0.8625, but with any other final decimal fraction, then the resulting an irreducible rational fraction would also have this property. In other words, the decomposition of the denominator b into prime factors could only include prime numbers 2 and 5, since b is a divisor of some power of 10, and . This circumstance turns out to be decisive, namely, the following general statement holds:
An irreducible rational fraction has a finite decimal representation if and only if the number b does not have prime divisors, personal from 2 and 5.
Note that in this case b does not have to have both 2 and 5 among its prime divisors: it can be divisible by only one of them or not divisible by them at all. For example,
here b is equal to 25, 16, and 1, respectively. The essential thing is that b has no other divisors other than 2 and 5.
The above sentence contains an expression if and only if. So far, we have only proved the part that applies to turnover only then. It was we who showed that the expansion of a rational number into a decimal fraction will be finite only if b has no prime divisors other than 2 and 5.
(In other words, if b is divisible by a prime number other than 2 and 5, then the irreducible fraction has no final decimal expression.)
The part of the sentence that refers to the word then states that if the integer b has no other prime divisors f other than 2 and 5, then an irreducible rational fraction can be represented by a finite decimal fraction. In order to prove this, we must take an arbitrary irreducible rational fraction, for which b has no other prime divisors except 2 and 5, and make sure that the corresponding decimal fraction is finite. Let's consider an example first. Let be
![]()
To obtain a decimal expansion, we convert this fraction into a fraction whose denominator is an integer power of ten. This can be achieved by multiplying the numerator and denominator by:
The above argument can be extended to the general case as follows. Suppose b is of the form , where the type is non-negative integers (i.e., positive numbers or zero). Two cases are possible: either less than or equal (this condition is written ), or greater (which is written ). When we multiply the numerator and denominator of the fraction by
Since the integer is not negative (i.e., positive or equal to zero), then, and therefore, a is a positive integer. Let . Then
In this article, we will understand what a decimal fraction is, what features and properties it has. Go! 🙂
The decimal fraction is a special case of ordinary fractions (in which the denominator is a multiple of 10).
Definition
Decimals are fractions whose denominators are numbers consisting of one and a certain number of zeros following it. That is, these are fractions with a denominator of 10, 100, 1000, etc. Otherwise, a decimal fraction can be characterized as a fraction with a denominator of 10 or one of the powers of ten.
Fraction examples:
, ,
A decimal fraction is written differently than a common fraction. Operations with these fractions are also different from operations with ordinary ones. The rules for operations on them are to a large extent close to the rules for operations on integers. This, in particular, determines their relevance in solving practical problems.
Representation of a fraction in decimal notation
There is no denominator in the decimal notation, it displays the number of the numerator. In general, decimal fractions are written as follows:
where X is the integer part of the fraction, Y is its fractional part, "," is the decimal point.
For the correct representation of an ordinary fraction as a decimal, it is required that it be correct, that is, with a highlighted integer part (if possible) and a numerator that is less than the denominator. Then, in decimal notation, the integer part is written before the decimal point (X), and the numerator of the ordinary fraction is written after the decimal point (Y).
If the numerator represents a number with a number of digits less than the number of zeros in the denominator, then in the Y part the missing number of digits in the decimal notation is filled with zeros in front of the numerator digits.
Example: ![]()
If the ordinary fraction is less than 1, i.e. does not have an integer part, then 0 is written in decimal form for X.
In the fractional part (Y), after the last significant (other than zero) digit, an arbitrary number of zeros can be entered. It does not affect the value of the fraction. And vice versa: all zeros at the end of the fractional part of the decimal fraction can be omitted.
Reading decimals
Part X is read in the general case as follows: "X integers."
The Y part is read according to the number in the denominator. For the denominator 10, you should read: "Y tenths", for the denominator 100: "Y hundredths", for the denominator 1000: "Y thousandths" and so on ... 😉
Another approach to reading is considered more correct, based on counting the number of digits of the fractional part. To do this, you need to understand that the fractional digits are located in a mirror image with respect to the digits of the integer part of the fraction.
Names for correct reading are given in the table:

Based on this, the reading should be based on the correspondence to the name of the category of the last digit of the fractional part.
- 3.5 reads "three point five"
- 0.016 reads like "zero point sixteen thousandths"
Converting an arbitrary ordinary fraction to a decimal
If the denominator of an ordinary fraction is 10 or some power of ten, then the fraction is converted as described above. In other situations, additional transformations are needed.
There are 2 ways to translate.
The first way of translation
The numerator and denominator must be multiplied by such an integer that the denominator is 10 or one of the powers of ten. And then the fraction is represented in decimal notation.
This method is applicable for fractions, the denominator of which is decomposed only into 2 and 5. So, in the previous example ![]() . If there are other prime factors in the expansion (for example, ), then you will have to resort to the 2nd method.
. If there are other prime factors in the expansion (for example, ), then you will have to resort to the 2nd method.
The second way of translation
The 2nd method is to divide the numerator by the denominator in a column or on a calculator. The integer part, if any, is not involved in the transformation.
The long division rule that results in a decimal fraction is described below (see Dividing Decimals).
Convert decimal to ordinary
To do this, its fractional part (to the right of the comma) should be written as a numerator, and the result of reading the fractional part should be written as the corresponding number in the denominator. Further, if possible, you need to reduce the resulting fraction.
![]()
End and Infinite Decimal
The decimal fraction is called final, the fractional part of which consists of a finite number of digits.
All the above examples contain exactly the final decimal fractions. However, not every ordinary fraction can be represented as a final decimal. If the 1st translation method for a given fraction is not applicable, and the 2nd method demonstrates that the division cannot be completed, then only an infinite decimal fraction can be obtained.
It is impossible to write an infinite fraction in its full form. In an incomplete form, such fractions can be represented:
- as a result of reduction to the desired number of decimal places;
- in the form of a periodic fraction.
A fraction is called periodic, in which, after the decimal point, an infinitely repeating sequence of digits can be distinguished.
The remaining fractions are called non-periodic. For non-periodic fractions, only the 1st representation method (rounding) is allowed.
An example of a periodic fraction: 0.8888888 ... There is a repeating figure 8 here, which, obviously, will be repeated indefinitely, since there is no reason to assume otherwise. This number is called fraction period.
Periodic fractions are pure and mixed. A decimal fraction is pure, in which the period begins immediately after the decimal point. A mixed fraction has 1 or more digits before the decimal point.
54.33333 ... - periodic pure decimal fraction
2.5621212121 ... - periodic mixed fraction
Examples of writing infinite decimals:
The 2nd example shows how to properly form a period in a periodic fraction.
Converting periodic decimals to ordinary
To convert a pure periodic fraction into an ordinary period, write it in the numerator, and write in the denominator a number consisting of nines in an amount equal to the number of digits in the period.
![]()
A mixed recurring decimal is translated as follows:
- you need to form a number consisting of the number after the decimal point before the period, and the first period;
- from the resulting number subtract the number after the decimal point before the period. The result will be the numerator of an ordinary fraction;
- in the denominator, you need to enter a number consisting of the number of nines equal to the number of digits of the period, followed by zeros, the number of which is equal to the number of digits of the number after the decimal point before the 1st period.
![]()
![]()
Decimal Comparison
Decimal fractions are compared initially by their whole parts. The larger is the fraction that has the larger integer part.
If the integer parts are the same, then the digits of the corresponding digits of the fractional part are compared, starting from the first (from the tenths). The same principle applies here: the larger of the fractions, which has a larger rank of tenths; if the tenths digits are equal, the hundredths digits are compared, and so on.
Insofar as
![]() , since with equal integer parts and equal tenths in the fractional part, the 2nd fraction has more hundredths.
, since with equal integer parts and equal tenths in the fractional part, the 2nd fraction has more hundredths.
Adding and subtracting decimals
Decimals are added and subtracted in the same way as whole numbers, writing the corresponding digits one under the other. To do this, you need to have decimal points under each other. Then the units (tens, etc.) of the integer part, as well as the tenths (hundredths, etc.) of the fractional part will match. The missing digits of the fractional part are filled with zeros. Directly The process of addition and subtraction is carried out in the same way as for integers.
Decimal multiplication
To multiply decimal fractions, you need to write them one under the other, aligned with the last digit and not paying attention to the location of the decimal points. Then you need to multiply the numbers in the same way as when multiplying integers. After receiving the result, you should recalculate the number of digits after the decimal point in both fractions and separate the total number of fractional digits in the resulting number with a comma. If there are not enough digits, they are replaced by zeros.

Multiplying and dividing decimals by 10 n
These actions are simple and come down to moving the decimal point. P When multiplying, the comma is moved to the right (the fraction increases) by the number of digits equal to the number of zeros in 10 n, where n is an arbitrary integer power. That is, a certain number of digits are transferred from the fractional part to the integer. When dividing, respectively, the comma is transferred to the left (the number decreases), and some of the digits are transferred from the integer part to the fractional part. If there are not enough digits to transfer, then the missing digits are filled with zeros.
Dividing a decimal and an integer by an integer and a decimal
Dividing a decimal by an integer is the same as dividing two integers. Additionally, only the position of the decimal point must be taken into account: when demolishing the digit of the digit followed by a comma, it is necessary to put a comma after the current digit of the generated answer. Then you need to keep dividing until you get zero. If there are not enough signs in the dividend for complete division, zeros should be used as them.

Similarly, 2 integers are divided into a column if all the digits of the dividend have been demolished, and the full division has not yet been completed. In this case, after the demolition of the last digit of the dividend, a decimal point is placed in the resulting answer, and zeros are used as the demolished digits. Those. the dividend here, in fact, is represented as a decimal fraction with a zero fractional part.

To divide a decimal fraction (or an integer) by a decimal number, it is necessary to multiply the dividend and the divisor by the number 10 n, in which the number of zeros is equal to the number of digits after the decimal point in the divisor. In this way, they get rid of the decimal point in the fraction by which you want to divide. Further, the division process is the same as described above.
Graphical representation of decimals
Graphically, decimal fractions are represented by means of a coordinate line. For this, single segments are additionally divided into 10 equal parts, just as centimeters and millimeters are deposited on a ruler at the same time. This ensures that decimals are displayed accurately and can be compared objectively.
In order for the longitudinal divisions on single segments to be the same, one should carefully consider the length of the single segment itself. It should be such that the convenience of additional division can be ensured.