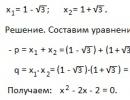Classic probability of an event. Probability theory. Probability of an event, random events (probability theory). Independent and incompatible events in probability theory. Solve the addition of probabilities problem yourself, and then look at the solution
1. Presentation of the main theorems and probability formulas: addition theorem, conditional probability, multiplication theorem, independence of events, total probability formula.
Goals: creating favorable conditions for introducing the concept of probability of an event; familiarity with the basic theorems and formulas of probability theory; introduce the total probability formula.
Progress of the lesson:
Random experiment (experience) is a process in which different outcomes are possible, and it is impossible to predict in advance what the outcome will be. Possible mutually exclusive outcomes of an experiment are called its elementary events . We denote the set of elementary events by W.
Random event is an event about which it is impossible to say in advance whether it will occur as a result of experience or not. Each random event A that occurred as a result of an experiment can be associated with a group of elementary events from W. The elementary events included in this group are called favorable for the occurrence of event A.
The set W can also be considered as a random event. Since it includes all elementary events, it will certainly occur as a result of experience. Such an event is called reliable .
If for a given event there are no favorable elementary events from W, then it cannot occur as a result of the experiment. Such an event is called impossible.
Events are called equally possible , if the test results in equal opportunity for these events to occur. Two random events are called opposite , if as a result of the experiment one of them occurs if and only if the other does not occur. The event opposite to event A is denoted by .
Events A and B are called incompatible , if the appearance of one of them excludes the appearance of the other. Events A 1, A 2, ..., A n are called pairwise incompatible, if any two of them are inconsistent. Events A 1, A 2, ..., An form a complete system of pairwise incompatible events , if one and only one of them is sure to occur as a result of the test.
The sum (union) of events A 1, A 2, ..., A n is called such an event C, which consists in the fact that at least one of the events A 1, A 2, ..., A n occurs. The sum of events is denoted as follows:
C = A 1 +A 2 +…+A n.
The product (intersection) of events A 1, A 2, ..., A n is called such an event P, which consists in the fact that all events A 1, A 2, ..., A n occurred simultaneously. The production of events is indicated
The probability P(A) in probability theory appears as numerical characteristic the degree of possibility of the occurrence of any specific random event A when tests are repeated many times.
Let's say that in 1000 throws of a die, the number 4 appears 160 times. The ratio 160/1000 = 0.16 shows the relative frequency of the number 4 in a given series of tests. In a more general case frequency of a random event And when conducting a series of experiments, the ratio of the number of experiments in which a given event occurred to the total number of experiments is called:
where P*(A) is the frequency of event A; m is the number of experiments in which event A occurred; n- total number experiments.
The probability of a random event And they call a constant number around which the frequencies of a given event are grouped as the number of experiments increases ( statistical determination of the probability of an event ). The probability of a random event is denoted by P(A).
Naturally, no one will ever be able to perform an unlimited number of tests in order to determine the probability. There is no need for this. In practice, the frequency of an event can be taken as probability at large number tests. For example, from the statistical patterns of birth established over many years of observation, the probability of the event that the newborn will be a boy is estimated at 0.515.
If during the test there are no reasons due to which one random event would appear more often than others ( equally possible events), the probability can be determined based on theoretical considerations. For example, let us find out in the case of tossing a coin the frequency of the coat of arms falling out (event A). different experimenters over several thousand tests have shown that the relative frequency of such an event takes values close to 0.5. considering that the appearance of the coat of arms and opposite side coins (event B) are equally possible events; if the coin is symmetrical, the judgment P(A) = P(B) = 0.5 could be made without determining the frequency of these events. Based on the concept of “equal possibility” of events, another definition of probability is formulated.
Let the event A under consideration occur in m cases, which are called favorable to A, and not occur in the remaining n-m, unfavorable to A.
Then the probability of event A is equal to the ratio of the number of elementary events favorable to it to their total number(classical definition of the probability of an event):
where m is the number of elementary events favorable to event A; n - Total number of elementary events.
Let's look at a few examples:
Example #1:An urn contains 40 balls: 10 black and 30 white. Find the probability that a ball chosen at random will be black.
The number of favorable cases is equal to the number of black balls in the urn: m = 10. The total number of equally possible events (taking out one ball) is equal to the total number of balls in the urn: n = 40. These events are inconsistent, since one and only one ball is taken out. P(A) = 10/40 = 0.25
Example #2:Find the probability of getting an even number when throwing a die.
When throwing a dice, six equally possible incompatible events occur: the appearance of one number: 1,2,3,4,5 or 6, i.e. n = 6. favorable cases are the occurrence of one of the numbers 2,4 or 6: m = 3. the desired probability P(A) = m/N = 3/6 = ½.
As we see from the definition of the probability of an event, for all events
0 < Р(А) < 1.
Obviously, the probability of a certain event is 1, the probability of an impossible event is 0.
Probability addition theorem: the probability of the occurrence of one (no matter which) event from several incompatible events is equal to the sum of their probabilities.
For two incompatible events A and B, the probabilities of these events are equal to the sum of their probabilities:
P(A or B) = P(A) + P(B).
Example #3:find the probability of getting 1 or 6 when throwing a die.
Events A (rolling 1) and B (rolling 6) are equally possible: P(A) = P(B) = 1/6, therefore P(A or B) = 1/6 + 1/6 = 1/3
The addition of probabilities is valid not only for two, but also for any number of incompatible events.
Example #4:There are 50 balls in the urn: 10 white, 20 black, 5 red and 15 blue. Find the probability of a white, or black, or red ball appearing during a single operation of removing a ball from the urn.
The probability of drawing the white ball (event A) is P(A) = 10/50 = 1/5, the black ball (event B) is P(B) = 20/50 = 2/5 and the red ball (event C) is P (C) = 5/50 = 1/10. From here, using the formula for adding probabilities, we get P(A or B or C) = P(A) + P(B) = P(C) = 1/5 + 2/5 + 1/10 = 7/10
The sum of the probabilities of two opposite events, as follows from the theorem of addition of probabilities, is equal to one:
P(A) + P() = 1
In the above example, taking out a white, black and red ball will be the event A 1, P(A 1) = 7/10. The opposite event of 1 is drawing the blue ball. Since there are 15 blue balls, and the total number of balls is 50, we get P(1) = 15/50 = 3/10 and P(A) + P() = 7/10 +3/10 = 1.
If events A 1, A 2, ..., A n form a complete system of pairwise incompatible events, then the sum of their probabilities is equal to 1.
In general, the probability of the sum of two events A and B is calculated as
P(A+B) = P(A) + P(B) - P(AB).
Probability multiplication theorem:
Events A and B are called independent , if the probability of occurrence of event A does not depend on whether event B occurred or not, and vice versa, the probability of occurrence of event B does not depend on whether event A occurred or not.
The probability of joint occurrence of independent events is equal to the product of their probabilities. For two events P(A and B)=P(A)·P(B).
Example: One urn contains 5 black and 10 white balls, the other contains 3 black and 17 white balls. Find the probability that when balls are first drawn from each urn, both balls will be black.
Solution: the probability of drawing a black ball from the first urn (event A) is P(A) = 5/15 = 1/3, a black ball from the second urn (event B) is P(B) = 3/20
P(A and B)=P(A)·P(B) = (1/3)(3/20) = 3/60 = 1/20.
In practice, the probability of event B often depends on whether some other event A occurred or not. In this case they talk about conditional probability , i.e. the probability of event B given that event A occurs. Conditional probability is denoted by P(B/A).
In order to quantitatively compare events with each other according to the degree of their possibility, obviously, it is necessary to associate a certain number with each event, which is greater, the more possible the event is. We will call this number the probability of an event. Thus, probability of an event is a numerical measure of the degree of objective possibility of this event.
The first definition of probability should be considered the classical one, which arose from the analysis of gambling and was initially applied intuitively.
The classical method of determining probability is based on the concept of equally possible and incompatible events, which are the outcomes of a given experience and form a complete group of incompatible events.
Most simple example equally possible and incompatible events forming a complete group is the appearance of one or another ball from an urn containing several balls of the same size, weight and other tangible characteristics, differing only in color, thoroughly mixed before removal.
Therefore, a test whose outcomes form a complete group of incompatible and equally possible events is said to be reducible to a pattern of urns, or a pattern of cases, or fits into the classical pattern.
Equally possible and incompatible events that make up a complete group will be called simply cases or chances. Moreover, in each experiment, along with cases, more complex events can occur.
Example: When throwing a dice, along with the cases A i - the loss of i-points on the upper side, we can consider such events as B - the loss of an even number of points, C - the loss of a number of points that are a multiple of three...
In relation to each event that can occur during the experiment, cases are divided into favorable, in which this event occurs, and unfavorable, in which the event does not occur. In the previous example, event B is favored by cases A 2, A 4, A 6; event C - cases A 3, A 6.
Classical probability the occurrence of a certain event is called the ratio of the number of cases favorable to the occurrence of this event to the total number of equally possible, incompatible cases that make up the complete group in a given experiment:
Where P(A)- probability of occurrence of event A; m- the number of cases favorable to event A; n- total number of cases.
Examples:
1) (see example above) P(B)= , P(C) =.
2) The urn contains 9 red and 6 blue balls. Find the probability that one or two balls drawn at random will turn out to be red.
A- a red ball drawn at random:
m= 9, n= 9 + 6 = 15, P(A)=
B- two red balls drawn at random:
The following properties follow from the classical definition of probability (show yourself):
1) The probability of an impossible event is 0;
2) The probability of a reliable event is 1;
3) The probability of any event lies between 0 and 1;
4) The probability of an event opposite to event A,
The classic definition of probability assumes that the number of outcomes of a trial is finite. In practice, very often there are tests, the number of possible cases of which is infinite. Besides, weak side The classical definition is that very often it is impossible to represent the result of a test in the form of a set of elementary events. It is even more difficult to indicate the reasons for considering the elementary outcomes of a test to be equally possible. Usually, the equipossibility of elementary test outcomes is concluded from considerations of symmetry. However, such tasks are very rare in practice. For these reasons, along with the classical definition of probability, other definitions of probability are also used.
Statistical probability event A is the relative frequency of occurrence of this event in the tests performed:
where is the probability of occurrence of event A;
Relative frequency of occurrence of event A;
The number of trials in which event A appeared;
Total number of tests.
Unlike classical probability, statistical probability is an experimental characteristic.
Example: To control the quality of products from a batch, 100 products were selected at random, among which 3 products turned out to be defective. Determine the probability of marriage.
The statistical method of determining probability is applicable only to those events that have the following properties:
The events under consideration should be the outcomes of only those tests that can be reproduced an unlimited number of times under the same set of conditions.
Events must have statistical stability (or stability of relative frequencies). This means that in different series of tests the relative frequency of the event changes little.
The number of trials resulting in event A must be quite large.
It is easy to verify that the properties of probability arising from the classical definition are also preserved in the statistical definition of probability.
It is clear that each event has a varying degree of possibility of its occurrence (its implementation). In order to quantitatively compare events with each other according to the degree of their possibility, obviously, it is necessary to associate a certain number with each event, which is greater, the more possible the event is. This number is called the probability of an event.
Probability of event– is a numerical measure of the degree of objective possibility of the occurrence of this event.
Consider a stochastic experiment and a random event A observed in this experiment. Let's repeat this experiment n times and let m(A) be the number of experiments in which event A occurred.
Relation (1.1)
called relative frequency events A in the series of experiments performed.
It is easy to verify the validity of the properties:
if A and B are inconsistent (AB= ), then ν(A+B) = ν(A) + ν(B) (1.2)
The relative frequency is determined only after a series of experiments and, generally speaking, can vary from series to series. However, experience shows that in many cases, as the number of experiments increases, the relative frequency approaches a certain number. This fact of stability of the relative frequency has been repeatedly verified and can be considered experimentally established.
Example 1.19.. If you throw one coin, no one can predict which side it will land on top. But if you throw two tons of coins, then everyone will say that about one ton will fall up with the coat of arms, that is, the relative frequency of the coat of arms falling out is approximately 0.5.
If, with an increase in the number of experiments, the relative frequency of the event ν(A) tends to some fixed number, then it is said that event A is statistically stable, and this number is called the probability of event A.
Probability of the event A some fixed number P(A) is called, to which the relative frequency ν(A) of this event tends as the number of experiments increases, that is,
This definition is called statistical determination of probability .
Let's consider a certain stochastic experiment and let the space of its elementary events consist of a finite or infinite (but countable) set of elementary events ω 1, ω 2, …, ω i, …. Let us assume that each elementary event ω i is assigned a certain number - р i, characterizing the degree of possibility of the occurrence of a given elementary event and satisfying the following properties:
This number p i is called probability of an elementary eventωi.
Let now A be a random event observed in this experiment, and let it correspond to a certain set
In this setting probability of an event A call the sum of the probabilities of elementary events favoring A(included in the corresponding set A):
The probability introduced in this way has the same properties as the relative frequency, namely:
And if AB = (A and B are incompatible),
then P(A+B) = P(A) + P(B)
Indeed, according to (1.4)
In the last relation we took advantage of the fact that not a single elementary event can favor two incompatible events at the same time.
We especially note that probability theory does not indicate methods for determining p i; they must be sought for practical reasons or obtained from a corresponding statistical experiment.
As an example, consider the classical scheme of probability theory. To do this, consider a stochastic experiment, the space of elementary events of which consists of a finite (n) number of elements. Let us additionally assume that all these elementary events are equally possible, that is, the probabilities of elementary events are equal to p(ω i)=p i =p. It follows that
Example 1.20. When throwing a symmetrical coin, getting heads and tails are equally possible, their probabilities are equal to 0.5.
Example 1.21. When throwing a symmetrical die, all faces are equally possible, their probabilities are equal to 1/6.
Now let event A be favored by m elementary events, they are usually called outcomes favorable to event A. Then
Received classical definition of probability: the probability P(A) of event A is equal to the ratio of the number of outcomes favorable to event A to the total number of outcomes
Example 1.22. The urn contains m white balls and n black balls. What is the probability of drawing a white ball?
Solution. The total number of elementary events is m+n. They are all equally probable. Favorable event A of which m. Hence, .
The following properties follow from the definition of probability:
Property 1. The probability of a reliable event is equal to one.
Indeed, if the event is reliable, then every elementary outcome of the test favors the event. In this case t=p, hence,
P(A)=m/n=n/n=1.(1.6)
Property 2. The probability of an impossible event is zero.
Indeed, if an event is impossible, then none of the elementary outcomes of the test favor the event. In this case T= 0, therefore, P(A)=m/n=0/n=0. (1.7)
Property 3.The probability of a random event is a positive number between zero and one.
Indeed, a random event favors only a part of the total number of elementary outcomes of the test. That is, 0≤m≤n, which means 0≤m/n≤1, therefore, the probability of any event satisfies the double inequality 0≤ P(A) ≤1. (1.8)
Comparing the definitions of probability (1.5) and relative frequency (1.1), we conclude: definition of probability does not require testing to be carried out in reality; the definition of relative frequency assumes that tests were actually carried out. In other words, the probability is calculated before the experiment, and the relative frequency - after the experiment.
However, calculating probability requires preliminary information about the number or probabilities of elementary outcomes favorable for a given event. In the absence of such preliminary information, empirical data are used to determine the probability, that is, the relative frequency of the event is determined based on the results of a stochastic experiment.
Example 1.23. Technical control department discovered 3 non-standard parts in a batch of 80 randomly selected parts. Relative frequency of occurrence of non-standard parts r(A)= 3/80.
Example 1.24. According to the purpose.produced 24 shot, and 19 hits were recorded. Relative target hit rate. r(A)=19/24.
Long-term observations have shown that if experiments are carried out under identical conditions, in each of which the number of tests is sufficiently large, then the relative frequency exhibits the property of stability. This property is that in different experiments the relative frequency changes little (the less, the more tests are performed), fluctuating around a certain constant number. It turned out that this constant number can be taken as an approximate value of the probability.
The relationship between relative frequency and probability will be described in more detail and more precisely below. Now let us illustrate the property of stability with examples.
Example 1.25. According to Swedish statistics, the relative frequency of births of girls for 1935 by month is characterized by the following numbers (the numbers are arranged in order of months, starting with January): 0,486; 0,489; 0,490; 0.471; 0,478; 0,482; 0.462; 0,484; 0,485; 0,491; 0,482; 0,473
The relative frequency fluctuates around the number 0.481, which can be taken as an approximate value for the probability of having girls.
Note that statistical data from different countries give approximately the same relative frequency value.
Example 1.26. Coin tossing experiments were carried out many times, in which the number of appearances of the “coat of arms” was counted. The results of several experiments are shown in the table.
In economics, as in other areas of human activity or in nature, we constantly have to deal with events that cannot be accurately predicted. Thus, the sales volume of a product depends on demand, which can vary significantly, and on a number of other factors that are almost impossible to take into account. Therefore, when organizing production and carrying out sales, you have to predict the outcome of such activities on the basis of either your own previous experience, or similar experience of other people, or intuition, which to a large extent also relies on experimental data.
In order to somehow evaluate the event in question, it is necessary to take into account or specially organize the conditions in which this event is recorded.
The implementation of certain conditions or actions to identify the event in question is called experience or experiment.
The event is called random, if as a result of experience it may or may not occur.
The event is called reliable, if it necessarily appears as a result of a given experience, and impossible, if it cannot appear in this experience.
For example, snowfall in Moscow on November 30 is a random event. The daily sunrise can be considered a reliable event. Snowfall at the equator can be considered an impossible event.
One of the main tasks in probability theory is the task of determining a quantitative measure of the possibility of an event occurring.
Algebra of events
Events are called incompatible if they cannot be observed together in the same experience. Thus, the presence of two and three cars in one store for sale at the same time are two incompatible events.
Amount events is an event consisting of the occurrence of at least one of these events
An example of the sum of events is the presence of at least one of two products in the store.
The work events is an event consisting of the simultaneous occurrence of all these events
An event consisting of the appearance of two goods in a store at the same time is a product of events: - the appearance of one product, - the appearance of another product.
Events form a complete group of events if at least one of them is sure to occur in experience.
Example. The port has two berths for receiving ships. Three events can be considered: - the absence of ships at the berths, - the presence of one ship at one of the berths, - the presence of two ships at two berths. These three events form a complete group of events.
Opposite two unique possible events that form a complete group are called.
If one of the events that is opposite is denoted by , then the opposite event is usually denoted by .
Classical and statistical definitions of event probability
Each of the equally possible results of tests (experiments) is called an elementary outcome. They are usually designated by letters. For example, a die is thrown. There can be a total of six elementary outcomes based on the number of points on the sides.
From elementary outcomes you can create a more complex event. Thus, the event of an even number of points is determined by three outcomes: 2, 4, 6.
A quantitative measure of the possibility of the occurrence of the event in question is probability.
The most widely used definitions of the probability of an event are: classic And statistical.
The classical definition of probability is associated with the concept of a favorable outcome.
The outcome is called favorable to a given event if its occurrence entails the occurrence of this event.
In the above example, the event in question—an even number of points on the rolled side—has three favorable outcomes. IN in this case known and general
number of possible outcomes. This means that the classical definition of the probability of an event can be used here.
Classic definition equals the ratio of the number of favorable outcomes to the total number of possible outcomes
where is the probability of the event, is the number of outcomes favorable to the event, is the total number of possible outcomes.
In the considered example
The statistical definition of probability is associated with the concept of the relative frequency of occurrence of an event in experiments.
The relative frequency of occurrence of an event is calculated using the formula
where is the number of occurrences of an event in a series of experiments (tests).
Statistical definition. The probability of an event is the number around which the relative frequency stabilizes (sets) with an unlimited increase in the number of experiments.
In practical problems, the probability of an event is taken to be the relative frequency for a sufficiently large number of trials.
From these definitions of the probability of an event it is clear that the inequality is always satisfied
To determine the probability of an event based on formula (1.1), combinatorics formulas are often used, which are used to find the number of favorable outcomes and the total number of possible outcomes.